is tan an odd function|Iba pa : Tuguegarao A function is odd if f (−x) = −f (x) f ( - x) = - f ( x). Tap for more steps. The function is odd. Free math problem solver answers your algebra, geometry, trigonometry, calculus, and . Hong Kong Race 10 SIGHT WINNER HANDICAP (CLASS 3) 1600m $2,050,000 ~ 08:45 PM. Tote. Live Tips
is tan an odd function,Learn the definition of odd function and how to use the identities of sin and cos to show that tan(x) = -tan(-x) for any x. See the answer and the explanation with examples on Socratic.For this problem #tan (-x)=-tan (x)#. For example . #tan (-45) = -1#. #tan .
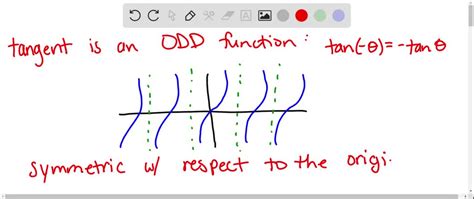
Is tangent even or odd? Answer: For a tangent function, f(−x) = −f(x), so tangent can be said to be an odd function. Go through the explanation to understand better. For this problem #tan (-x)=-tan (x)#. For example . #tan (-45) = -1#. #tan (45)=1#. hope that helped. Answer link. From the graph of tanx it can be seen that it is symmetric with respect to the origin. This .A function is odd if f (−x) = −f (x) f ( - x) = - f ( x). Tap for more steps. The function is odd. Free math problem solver answers your algebra, geometry, trigonometry, calculus, and .

Tangent is one of the six trigonometric functions that can be defined in terms of right triangles or unit circles. It is undefined at odd multiples of 90° and has a period .Trigonometric functions are examples of non-polynomial even (in the case of cosine) and odd (in the case of sine and tangent) functions. The properties of even and odd functions are useful in analyzing .The tangent function is an odd function because tan (-x) = -tan x. Tan x is not defined at values of x where cos x = 0. The graph of tan x has an infinite number of vertical asymptotes.
EVEN AND ODD TRIGONOMETRIC FUNCTIONS. An even function is one in which \(f(−x)=f(x)\). An odd function is one in which \(f(−x)=−f(x)\). Cosine and secant are .
How To Determine Trig Function Values Based Upon Whether The Function Is Odd Or Even? Determine each function value. If cos(x) = 0.5, then cos(-x) = ___. If sin(x) = 0.15, then sin(-x) = ___. If tan(-x) = . When we are given the equation of a function f(x), we can check whether the function is even, odd, or neither by evaluating f(-x). If we get an expression that is .
Furthermore, the tangent is an odd function, since it is symmetric with respect to the origin (see Observation even-odd): \[\label{EQ:tan-odd} \boxed{\tan(-x)=-\tan(x)} \] Recall from section . tanx is odd. If function is even, then f(-x) = f(x) If odd, f(-x) = -f(x). Recall that tanx = (sinx)/(cosx) f(-x) = (sin(-x))/(cos(-x)) = (-sin(x))/(cos(x)) = -tan(x .The tangent function is not defined at odd multiples of π/2 as the length of the base in a right triangle is 0 and cos x = 0 when x = kπ/2, where k is an odd integer. Hence, the domain of tan x is all real numbers except .The product of any two odd functions is an even function. The quotient of any 2 odd functions is an even function. Composition: The composition of any 2 odd functions is odd. The derivative of any given odd function is even in nature. The integral of any given odd function from the limits – A to + A is 0.

Inverse Tangent is Odd Function. This article is complete as far as it goes, but it could do with expansion. In particular: Expand for tan−1 tan − 1 on complex plane, include this as a corollary. You can help Pr∞fWiki P r ∞ f W i k i by adding this information. To discuss this page in more detail, feel free to use the talk page.
hope that helped. From the graph of tanx it can be seen that it is symmetric with respect to the origin. This tells us that it is an odd function . We can also check algebraically . Algebraically , if a function is: odd: f (-x) = -f (x) even: f (x)=f (-x) For this problem tan (-x)=-tan (x) For example . tan (-45) = -1 tan (45)=1 hope that .The sine function, then, is an odd function. We can test each of the six trigonometric functions in this fashion. . cosine, cosecant and secant, a revolution of one circle, or 2 π, 2 π, will result in the same outputs for these functions. And for tangent and cotangent, only a half a revolution will result in the same outputs. Other . Finding whether the inverse trigonometry function is odd or even. Let the function : (−,) → (−π 2, π 2): ( −,) → ( − π 2, π 2) be given by (u) = 2tan−1eu − π 2 ( u) = 2 tan − 1 e u − π 2, then is. (A) even and strictly increasing in (0,) ( 0,) (B) odd and strictly decreasing in (−,) ( −,) (C) odd and strictly .is tan an odd functionThe cosine function and all of its Taylor polynomials are even functions. In mathematics, an even function is a real function such that for every in its domain. Similarly, an odd function is a function such that for every in its domain. They are named for the parity of the powers of the power functions which satisfy each condition: the function .
is tan an odd function|Iba pa
PH0 · is tangent an odd function
PH1 · is tan x over y
PH2 · Iba pa